Hacking, I.
1980 “The Idea of Probable Inference: Neyman, Peirce and Braithwaite”, pp. 141-160 in D. H. Mellor (erectile dysfunction.), Science, Belief and Behavior: Essays in Honor of R.B. Braithwaite. Cambridge: Cambridge College Press.
Laudan, L.
1981 Science and Hypothesis: Historic Essays on Scientific Methodology. Dordrecht: ). Reidel.
Levi, I.
1980 “Induction as Self Correcting Based on Peirce”, pp. 127-140 in D. H. Mellor (erectile dysfunction.), Science, Belief and Behavior: Essays in Recognition of R.B. Braithwaite. Cambridge: Cambridge College Press.
Mayo, D.
1991 “Novel Evidence and Severe Tests”, Philosophy of Science. 58: 523-552.
— 1993 “The Exam of Experiment: C. S. Peirce and E. S. Pearson”, pp. 161-174 in E. C. Moore (erectile dysfunction.), Charles S. Peirce and also the Philosophy of Science. Tuscaloosa: College of Alabama Press.
— 1996 Error and also the Development of Experimental Understanding. The College of Chicago Press, Chicago.
— 2003 “Severe Testing like a Guide for Inductive Learning”, in H. Kyburg (erectile dysfunction.), Probability May be the Very Guide in Existence. Chicago: Open Court Press, pp. 89-117.
— 2004 “Evidence as Passing Severe Tests: Highly Probed versus. Highly Demonstrated” in P. Achinstein (erectile dysfunction.), Scientific Evidence. Johns Hopkins College Press.
Mayo, D. and Kruse, M.
2001 “Concepts of Inference as well as their Effects,” pp. 381-403 in Foundations of Bayesianism. D. Cornfield and J. Williamson (eds.), Dordrecht: Kluwer Academic Publishers.
Mayo, D. and Spanos, A.
2004 “Methodology used: Record Misspecification Testing” Philosophy of Science. Vol. II, PSA 2002, pp. 1007-1025.
— forthcoming “Severe Testing like a Fundamental Concept inside a Neyman-Pearson Theory of Induction”, The British Journal of Philosophy of Science .
Mayo, D. and Cox, D.R.
2005 “The Idea of Statistics because the ‘Frequentist’s’ Theory of Inductive Inference”, Institute of Mathematical Statistics (IMS) Lecture Notes-Monograph Series, Contributions towards the Second Lehmann Symposium. 2005.
Neyman, J. and Pearson, E.S.
1933 “Around the Problem of the very most Efficient Tests of Record Ideas”, in Philosophical Transactions from the Royal Society, A. 231, 289-337, as reprinted in J. Neyman and E.S. Pearson (1967), pp. 140-185.
— 1967 Joint Record Papers. Berkeley: College of California Press.
Niiniluoto, I.
1984 Is Science Progressive? Dordrecht: ). Reidel.
Peirce, C. S.
Collected Papers. Vols. I-Mire, C. Hartshorne and P. Weiss (eds.) (1931-1935). Vols. VII-VIII, A. Burks (erectile dysfunction.) (1958), Cambridge: Harvard College Press.
Popper, K.
1962 Conjectures and Refutations: the development of Scientific Understanding. Fundamental Books, New You are able to.
Rescher, N.
1978 Peirce’s Philosophy of Science: Critical Studies in the Theory of Induction and Scientific Method. Notre Dame: College of Notre Dame Press.
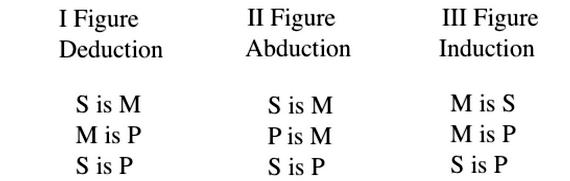
1. Other people who relate Peircean induction and Neyman-Pearson exams are Isaac Levi (1980 ) and Ian Hacking (1980 ). See also Mayo 1993 and 1996.
2. This statement of (b) is considered by Laudan because the strong thesis of self-correcting. A less strong thesis would replace (b) with (b’): science has approaches for figuring out unambiguously whether an alternate T’ is nearer to the reality than the usual refuted T.
3. When the p-value weren’t really small, then your difference could be considered statistically minor (generally small values are .1 or fewer).

We’d then regard H0 as in line with data x but we may decide to go further and see how big an elevated risk r which has therefore been eliminated with severity. We all do so by locating a risk increase, so that, Prob(d(x) d(x) risk increase r ) is high, say. Then your assertion: the danger increase r passes rich in severity, we’d argue.
If there have been a discrepancy from hypothesis H0 of r (or even more), then, rich in probability, 1-p. the information could be statistically significant at level p .
x isn’t statistically significant at level p .
Therefore, x is evidence than any discrepancy from H0 is under r .
For any general management of effect size. see Mayo and Spanos (forthcoming).
2005 Charles S. Peirce Society




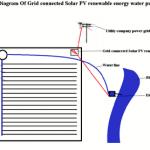 Grid connected pv system thesis proposal
Grid connected pv system thesis proposal Phd thesis proposal presentation ppt file
Phd thesis proposal presentation ppt file Samples of questionnaires for thesis proposal
Samples of questionnaires for thesis proposal The proposal of dissertation definition
The proposal of dissertation definition Like a winding sheet thesis proposal
Like a winding sheet thesis proposal